A. History and listening references
The purpose of this study was to determine, with listening
tests in an objective situation, what divergence from an optimum
frequency response curve in the bass range is inaudible. It was
also desired to determine some type of subjective description
of the "sound" from different divergencies from the
"optimum curves". This last task resulted in two additional
sets of curves. These two curves show how to minimise the subjective
distortion from a dynamical and a statical point
of view, despite greater measurable reduction in the bass output.
In the case when you, for one reason or another, want to design
a speaker system with a response curve that deviates from the
"inaudible curves" (e.g. to gain box size, efficiency
or power capacity), I would suggest a compromise between both
the dynamical and the statical characteristics.
(Except, of course, if you request a colouration from the speaker.)
Later experiments conducted with a large number of people have
shown that, to a certain extent, audiophiles prefer the
dynamical more than the statical qualities, while
the average listener seems to attach more importance to the statical
behaviour. Classically aligned vented boxes (flat curve down
to fh, then 24dB/octave rolloff thereafter) with a
40 Hz cut off frequency also manage well with the average listener.
However, audiophiles and live concert listeners often react to
the sharper cut off from the statical optimisation with
comments such as: "The bass is boxy, tired, undynamical,
unmusical, boomy, unnatural." and so on. On the other hand
the audiophiles and live concert listeners have less criticism
for the larger cut off range with dynamical optimisation.
Some have even said "More air, more distinct, cleaner bass
reproduction" about the audibly reduced but dynamically
optimised curves. The same curves that ordinary listeners call:
"The bass has lost weight, sounds cold, no depth",
and so on.
Until now all discussions have been about linear systems, which
means those that are undistorted. In reality, however, nonlinear
distortions provide a large part of the audible alteration of
the reproduced sound from commercially available loudspeakers.
(This will be discussed further in the text under the heading
"Statically optimised closed boxes".)
Conditions chosen for the original study (1981)
To be able to test the properties of human hearing of the
bass range in an objective way, it is necessary to give the person
in the test a reference to compare the deviations with.
If the experimental results shall be of any value, it is important
that the reference is as "true" as possible when creating
the conditions in audio reproduction. Therefore, the following
testing conditions were demanded:
1. All listening should be take place in an echo free
(anechoic) environment.
2. The reference reproduction chain should have a flat frequency
down to at least 12 Hz. This includes the entire chain
from microphones, via tape recorder and amplifier, to the monitor
speakers.
3. The musical/sound material to be used should vary over a wide
range from nearly completely statical sounds (e.g. the
lowest notes from large organs), to almost completely dynamical
bass sounds (e.g. symphonic bass drums, doors closed to tight
small rooms). About one hour of test material was collected for
the study.
4. The electronic filter box to be used for simulating the different
alignments should have complete flexibility to simulate all
possible alignments of both vented and closed boxes. A specification
of a S/N better than 100 dB and distortion less than 0.1% over
the 0-20000 Hz frequency range was also demanded. It may be stressed
that these specifications are not enough for all audio purposes,
but for a bass range only study the demands are lower.
The procedure
(1) First the echo free environment was to be established.
It is common that many actual echo free rooms have room influences as
high as 200 Hz. Then things get worse the lower in frequency
you go. What I needed was free field conditions down to 10 Hz,
which is much lower than a standard echo free room. The only
alternative was to use a a true free field! This can be found
on top of a high house with a steep roof ridge or high enough
up in the air to be able to disregard the ground reflection.
The choice was made to use a high tree, 12 metres up. The
distance between the loudspeakers and the test person was set
to 0.5-1 metres, and the intensity of the ground reflection was
measured to be 1/700 of the direct sound.
(2) Now the high specified reference equipment was to
be composed. Of these, the microphones where the least problematical.
Two measurement microphones were used, with a cut off
frequency of 0.2 Hz. The second link was the microphone
amplifier. It was built using discrete components and was
provided with an AC-coupling of 0.1 Hz. The tape recorder
that was chosen was a Technics. It could on the lowest speed
reach 8 Hz after a small modification. The range 10-20 Hz was
provided with a small lift. The power amplifier was DC-coupled.
Finally, the loudspeakers remained to be designed. The
demand for sound pressure was moderate, since the listening was
to take place at a short distance. A check of the recorded material
showed that the range below 25 Hz needed much less power capacity.
The choice was for 6.5" bass drivers in 48 litre boxes.
To get the required parameters in the easiest possible way, without
the use of too much equalisation correction, the cone mass was
much increased and the suspension slightly modified. With these
modifications the cut of frequency was 10 Hz. The range between
10 and 20 Hz showed a reduction complemented by the rise from
the tape recorder. The complete reproduction chain showed
a response within +/-1dB between 11 and 160 Hz. An upper range,
not of exceptionally high quality, was added in order to fulfill
the desire to have a "true" reference and had a response
of +/-2 to 3 dB up to 20kHz. This speaker was far from optimised
for normal music listening. First, it had a flat frequency in
a free field, second, the sensitivity was sensationally low (approximately
74 dB per W!) without a corresponding high power capacity (it
was 100 W). For this experiment, however, the speakers where
perfect.
(3) The music/sound program material was to be chosen,
and recorded. The recordings where made, with few exceptions,
in natural acoustical surroundings. Just about any instrument
with bass output was recorded, even those with their fundamental
tone in the higher bass range (e.g. kettledrums).
(4) The electronic box was fitted with four knobs representing
the coordinates of the pole pairs. It also had a three-way switch
to remove one pole pair or replace it with a single zero pole
(aperiodic response). Using the first, closed boxes could be
simulated, and with the single zero pole it was possible to simulate
dipole speakers.
B. The listening tests: optimum frequency response curves
The original purpose of the tests was only to obtain limits
of "allowed" frequency response deviations in the bass
range. It soon was apparent that, even if it was possible to
find these outer limits, it was not enough to stay within them!
Well, maybe not quite so surprising, since obviously very sharp
bends even within these limits may produce large group delays.
Anyway, it was a surprise as to what small deviations where audible.
It was necessary to establish a whole set of curves to get a
useful result, and this was obtained using seven curves. When
you stay on these curves, or between two of them, it is possible
to make the alignments "inaudible".
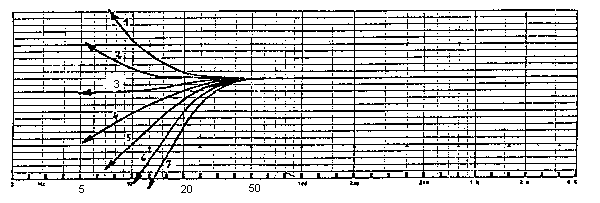
Optimum bass range frequency response curves (statically
& dynamically uncoloured). Allowable deviation from
any curve, but not outside curves 1-7, is as follows:
50 Hz: +1.0 / -0.5 dB, 40 Hz: +1.5 / -1.0 dB, 30 Hz: +1.5 / -2.0
dB, 20 Hz: +1.5 / -5.0 dB, 15 Hz: +2.0 / -10 dB, 10 Hz: +3.0
/ -15 dB, 7 Hz: +5.0 / -25 dB
C. The listening tests: semi-optimised frequency response
curves
As step 2 it was to be examined if the curves could be further
cut off if the variety of the test signals was reduced. It was
soon noted that it was possible to divide the music/sounds into
two main parts, placing completely different demands on the frequency
response curve. On the one hand, the dynamical test signals
(e.g. bass drums, stamping on a wooden floor, the closing of
doors, pizzicato on the double bass and similar sounds) were
seriously distorted by abrupt bends in the response curve. Then
again, it did not matter much if the entire bass range was tilted
down gently towards lower frequencies:
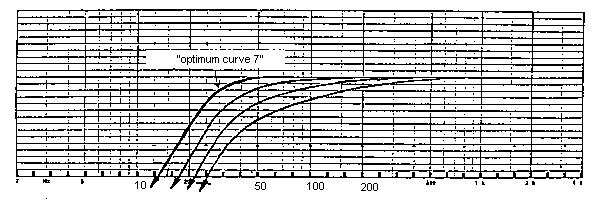
Dynamically optimised frequency response curves in the bass
range. Note: the upper thicker curve represents the sharpest
cut off that is acceptable both statically and dynamically.
On the other hand we have the statical signals (e.g.
the organ, the bass tuba, etc.). With these signals it was important
to keep a correct balance between the bass range as a whole (20-150)
and the upper range (150-20 000 Hz). It proved to be sonically
better to cut off rather sharply and equalise the remaining bass
with a lift in the curve just above the cutoff frequency.
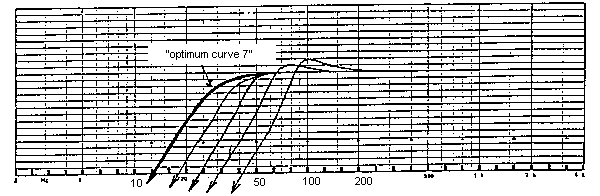
Statically optimised frequency response curves in the bass
range. Note: the upper/left thicker curve represents the sharpest
cut off that is acceptable both statically and dynamically.
When the cutoff frequency approaches 60 Hz a somewhat resonant
and boomy quality appeared, especially on the male voice, if
the remaining bass was elevated to compensate for the lost low
bass.
Later experiments have showed that this problem, due to unlinear
characteristics, is much worse from closed overemphasized boxes
than from vented boxes, where the rise is produced by the vent.
This may seem a little strange since the cutoff is much steeper
from the vented design, but the explanation comes later in the
text, see: "Unlinear properties of Closed boxes".
The semi optimised (not to be confused with optimum) curves
with different amounts of cutoff are like the "optimised
curves" presented by two set of curves, divided to the dynamically
and the statically optimised curves. In both cases
curve no. 7 (the sharpest cutoff that is acceptable both statically
and dynamically) is plotted as reference.
Closed boxes: Optimum curves
Here I have limited the set to three curves, each of which
is named after the driver resonance frequency in the box, 14,
20 and 33:

Examples of optimum frequency response curves for closed
boxes.
Curve "33" is the one that is closest to optimum
curve 7, and is well suited to most rooms.
(fo=33Hz, Q=0.707)
Curve "20" is adapted for a room of 20 square metres.
If the room is sealed and tight, the response is ruler flat down
to 0 Hz!
(fo=20Hz, Q=0.5)
Curve "14" is analogously adapted for a room of 40
square metres.
(fo=14Hz, Q=0.5)
Both of the last two curves represent an aperiodic low-frequency
closed box alignment (Qtc=0.5 and response is -6dB at resonance).
The drawback of closed boxes for these alignments is that they
need four times the cone area, or a longer excursion capability
(stroke), to be able to produce the same sound pressure with
the same distortion as vented boxes (one 8" vented driver
is approximately equal to two 12" drivers or one 15"
driver mounted in a closed box).
Dynamically optimised closed boxes
Here I will present some simple mathematical rules, rather
than frequency response curves. It should be sufficient to keep
the system Q on 25/fo. If you would like to have a cutoff higher
than 50 Hz, the Q should continue to fall below 0.50 according
to the formula. For fo at lower frequencies, Q should not be
higher than 0.707, and below 33 Hz the Q shall gradually approach
0.50, as in the optimum curves "20" and "14"
decribed above.
Statically optimised closed boxes
Here, too, some mathematics explains it best. If you chose
to cut higher than for the optimum curve 7, a sonic compensation
for lost bass is needed to increase what is left of the bass
range. A suitable choice of compensation is: Q=1/sqrt2*(fo/33)^0.65.
Higher fo frequencies than 85 Hz are not recommended under any
circumstances. Already at 85 Hz we need a compensation boost
of 3 dB at 100 Hz. and here we enter what has been implied earlier:
Nonlinear properties of closed boxes
I mentioned earlier that the ear, as opposed to what you might
initially believe, prefers the compensation that can be done
with statically optimised vented boxes over the same compensation
applied to closed boxes. There are two reasons for this.
Reason 1: The cones of closed boxes have their speed maximum
occurring in the frequency range, which is lifted in amplitude.
Vented boxes, on the other hand, have their speed minimum at
the lifted frequencies (if the helmholtz resonance of the box
has been used to create the lift). When designing for statically
optimised curves, this result in a problem for the closed box
- the amplitude and distortion maxima coincide for the closed
box, while the amplitude maximum coincides with the distortion
minimum for the vented box.
Reason 2: An elevation from a closed box exaggerates, because
of thermal reasons, more the louder you play. For a vented box,
however, the elevation will decrease with increased level, because
of thermal reasons and losses in the vent.
D. An attempt for a "general" optimisation for vented
and closed boxes
Firstly, I would like to say that this part of the report
has left the objective scientific world. This is about my own
personal tastes now. The following alignments are definitely
audible. You can still try to balance the faults in order to
find the best possible alignment, even for a less than ideal
response curve, if the circumstances preclude a design according
to the optimum curves. My personal experience is that a semi-optimised
vented box sounds best if you start with a 4th-order Butterworth
alignment, and lower the fh frequency by 20-25%. When
it comes to closed boxes, I am afraid that I have found that
the most classical of all the alignments, the one with Qtc=1/sqrt(2)=0.7071,
gives the best compromise between dynamical and statical
behaviour. Possibly still, a little lower Q value, like Q=0.65
may be preferred.
If the possibility arises, I recommend that everyone try to design
genuine "optimum curve" speakers. In comparison, the
semi-optimised designs (dynamically, statically
or something in between), appear to be of lower sonic and musical
quality for listeners with greater expectations.
E. Reservations
Several times in the text, the so called "optimum curves"
have been referred to as "inaudibly coloured" compared
to a flat response. I would especially like to point out the
following:
1. To achieve an unobjectionable bass response it is obvious
that the system must not have any of the potential flaws. Beyond
the demand to comply with the optimum curves, the system must
have a distortion well below the threshold of audibility at the
sound pressure levels to be used. In practice, distortion values
of 10-20% are common when playing at the level of a symphony
orchestra.
2. From a strict scientific point of view, the result of an experiment
is only valid under the conditions that prevailed during the
course of the experiment. Here it is primarily the program material
I am thinking of. It is possible that some other sounds might
further increase the demands on the frequency response curve.
Considering the wide range of sounds used for this study, I do
believe that only marginal adjustments, if any, would have to
be done. Maybe curve 1 and possibly curve 2 could be dispensed
with.
3. One must not forget that speakers are usually placed in rooms
and not in a free field. This causes the need to consider more
properties for loudspeaker design than what the curves show about
hearing. Firstly, there are two room-related properties that
have to be included in every serious loudspeaker project, and
these are:
a. The influence of the floor reflection
Depending on the woofers' height over the floor, the contribution
from the floor will be in phase with the direct radiation from
the driver up to a higher or lower frequency. Some examples are
shown below.
Height: 70 cm > 250 Hz (1st cancellation
at approximately 500 Hz).
Height: 30 cm > 600 Hz (1st cancellation
at approximately 1200 Hz).
Height: 10 cm > 1800 Hz (1st cancellation
at approximately 3600 Hz).
Below this frequency the level will be elevated approximately
2-6 dB compared to higher frequencies. To be more specific, the
level is elevated 6 dB when the reflection is in phase with the
direct radiation and, on an average, it is elevated 3 dB in the
higher range where the reflection appears in random phase.
Both the 6 dB increase at low frequencies and the 3 dB increase
at higher frequencies can be less depending on how elastic the
reflective surface is (for low frequencies) and how absorbing
it is (for high frequencies). Therefore, the interval is 2-6
dB.
b. The cavity effect of the room
Real rooms contribute with more bass increase than if they
had been a "theatre box" open in the front (this is
not a "Voice of the Theatre" speaker box, but the place
you sit in a theatre). In practice, there are of course many
variations between different listening rooms, but it is possible
to draw a "typical room curve". From it you can also
see some typical floor modifications.
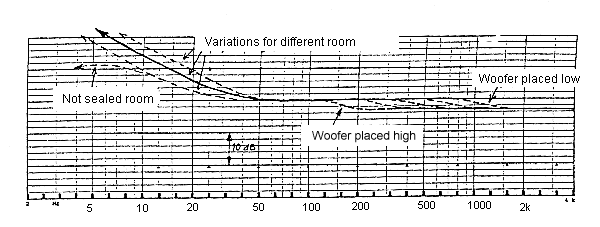
Comments: The influences of the standing waves in the room
are not included in these curves for several reasons. Firstly,
they belong to the listener's acoustic in the "theatre box".
Secondly, there are no "general standing waves"; all
rooms are unique. It should also be pointed out that a room-adapted
speaker will have an inverted frequency response compared with
these curves. You can also see that you do not have to worry
about the floor reflection if the woofer is placed low to the
ground and the midrange is placed high and the crossover point
is placed between the floor knees of the two drivers. You should,
however, see to it that the woofer has 2-4 dB less output in
its free field response, without the help from the floor.
4. Another important reservation is that you should not interpret
the "optimum curves" as being in any way absolute.
For example, only the cutoffs which can feasibly be obtained
with closed and vented boxes (and dipoles) have been simulated.
It may be possible that the ear would accept steeper cutoffs
than those shown. The curves shall not be seen as implying the
limit of the ear to hear deviations from the response.
Instead, they show the tolerance of the ear for different
bass alignments which it is possible to obtain with loudspeakers.
5. You should not forget that details like windows, pictures,
panels and such can begin to distort (rattle) at high levels.
In this case, the response down to at least 10-15 Hz will influence
the result.
In a closed room, the optimum response curves 4-7 should be chosen
for the most neutral sound, with the greatest safety margin before
the onset of audibly distorted sound. For listening outdoors,
the optimum response curves 1-3 are valid.




